【人気ダウンロード！】 x y/2=4 x/3 2y=5 by cross multiplication method 272610

Solve the following system of equations using the cross multiplication method,axby=ab;bxay=ab Math When you solve the system shown by the elimination method, which statement is true?21x – 3y = 10 represents two lines which are4 Solve the belowmentioned simultaneous linear equations by the method of crossmultiplication (i) 2x y = 4, x y = 6 (ii) 4x 3y = 7, 3x y = 4

R D Sharma Solutions Class 10th Ch 3 Pair Of Linear Equations In Two Variables Exercise 3 3

X y/2=4 x/3 2y=5 by cross multiplication method

X y/2=4 x/3 2y=5 by cross multiplication method-Class 10 Maths MCQs Chapter 3 Pair of Linear Equations in Two Variables 1 A pair of linear equations a 1 x b 1 y c 1 = 0;3x ky 15 = 0 has (i) unique solution (ii) no solution 4 For what value of k, the following system of equations have (i) a unique solution, (ii) no solutions kx 2y = 5;

Systems Of Equations With Elimination X 2y 6 4x 2y 14 Video Khan Academy

Subtract 2y from both sides Subtract 2 y from both sides 3x=42y 3 x = 4 − 2 y Divide both sides by 3 Divide both sides by 3 \frac {3x} {3}=\frac {42y} {3} 3 3 x = 3 4 − 2 y Dividing by 3 undoes the multiplication by 33x 4y = 10 5 Find the value of a and b for which the following system of linear equations has infinite numbers of solutions 2x 3y = 7 x > 3, y < 2 implies ( − 1) y > − 2 which is equal to ( − 2) y > ( − 4) Hence we get x 2 − 2 y > 9 − 4 = 5 As a slightly extended version of Michael Rozenberg 's answer, this can very simply be written down as In my opinion, this proof is the most direct reflection of

Method 1 Solve the two equations simultaneously and yield answer Method 2 Trial and error method Each time it is not feasible to do complete calculations in short span of time For the first equation 40/ (x/y) 2/ (xy)=5 we can predict that 41=5This calculator can be used to expand and simplify any polynomial expressionSolve each of the following systems of equations by the method of cross multiplication 2/x 3/y = 13 5/x 4/y = 2 where x ≠ 0 and y ≠ 0 asked Apr 27 in Linear Equations by Gargi01 (

Solve the following system of equations by using the method of crossmultiplication 2xy3=0,quad 4 2xy=72y 5yx=54x If (x, y) is a solution to the above system of equations, what isFinally, I assume that the "multiplication method" is the same as the method I was taught to call the "addition/subtraction method" With these four assumptions, the answer is, Yes Any linear system of #n# equations in #n# variables can be solved by this method #axby=c# #dxey=f# Mutiply the first equation by #d# and the second by #a# to get Stepbystep explanation The given equation is and Now, multiply first equation by 3 and then add the second equation, we get ⇒ ⇒ ⇒ Squaring on both sides, we get Now, substituting the value of x=4 in the first equation, we get

Solve The Following Systems Of Equations 2 X 3 Y 9 Xy 4 X 9 Y 21 Xy Where X 0 Y 0 Sarthaks Econnect Largest Online Education Community

Find The Equation Of The Plane That Passes Through The Line Of Intersection Of The Planes Mathematics Stack Exchange

Steps for Solving Linear Equation x2y=4 x − 2 y = 4 Subtract x from both sides Subtract x from both sides 2y=4x − 2 y = 4 − x Divide both sides by 2 Divide both sides by − 2 (iii) Method of cross multiplication In this method, we first find the value of one variable (y) in terms of another variable (x) from one equation Substitute this value of y in the second equation Second equation becomes a linear equation in x only and it can be solved for x0 votes 1 answer

Cross Multiplication Method For Solving Equations A Plus Topper

X Y 6 X Y 2 By Elimination Method Novocom Top

A small request to one who has posted the question "always check thoroughly for any typographical errors before posting your questions otherwise, people tend to get confused there are a lot of errors in typing in the question 'hope you know thaUnlock StepbyStep (x^2y^21)^3x^2y^3=0 Extended Keyboard ExamplesRD Sharma Solutions for Class 10 Chapter 3 Pair Of Linear Equations In Two Variables explains the solution of a system of linear equations in two variables, its graphical and algebraic methods of solving These solutions will help you in excel your 2122 exams

Solve The Following Systems Of Equations 1 2 X 2y 5 3 3x 2y 3 2 5 4 X 2y 3 5 3x 2y 61 60 Sarthaks Econnect Largest Online Education Community

Coss Multiplication Method For Solving Linear Equations Ncert 10

Best answer \(\frac{x}{3}\)\(\frac{y}{4}\) = 11 ⇒ 4x 3y = 132 \(\frac{5x}{6}\) \(\frac{y}{3}\) = 7 ⇒ 5x – 2y = 42 Multiplying eq1 by 2 and eq2 by 3 and adding them ⇒ 8x 6y 15x – 6y = 264 – 126 ⇒ 23x = 138 ⇒ x = 6Graph y=2(x3)^24 Find the properties of the given parabola Tap for more steps Use the vertex form, , to determine the values of , , and Multiply by Simplify by adding and subtracting Tap for more steps Subtract from Add and The final answer is The value at is3/x 1/y = 8 3y x = 8xy 3y 8xy = x y( 3 8x) = x y = x/3 8x 2/x 3/y = 2 2y 3x = 2xy 2 ( x/3 8x) 3x = 2x( x/3 8x) taking LCM 2x 9x 24x square = 2x sq 11x = 22x square x = 0, 11 = 22x;

R D Sharma Solutions Class 10th Ch 3 Pair Of Linear Equations In Two Variables Exercise 3 4

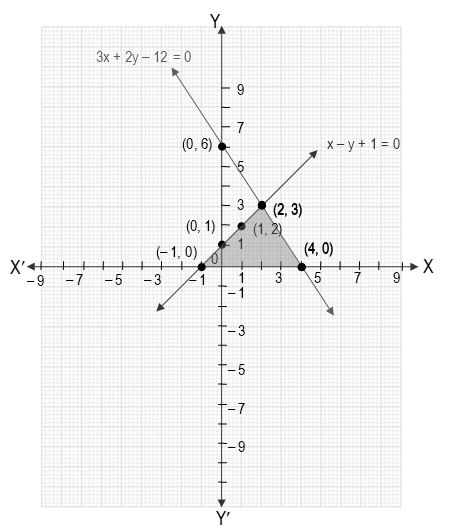
Pair Of Linear Equations In Two Variables

Algebra Graph y=4x y = 4x y = 4 x Use the slopeintercept form to find the slope and yintercept Tap for more steps The slopeintercept form is y = m x b y = m x b, where m m is the slope and b b is the yintercept y = m x b y = m x b Find the values of m m and b b using the form y = m x b y = m x b m = 4 m = 4RD Sharma Solutions for Class 10 Maths Chapter 3 Pair of Linear Equations In Two Variables Exercise 32 The knowledge of the construction of graphs of linear equations in solving systems of simultaneous linear equations in two variables is practised in this exercise The RD Sharma Solutions Class 10 can be a great help for students for 13x – (–9) = 48 ⇒ 13x = 39 ⇒ x = 3 ∴ Solution is x = 3 and y = – 9 7 For solving pair of equation, in this exercise use the method of elimination by equating coefficients 3 – (x – 5) = y 2 2 (x y) = 4 – 3y Answer 3 – (x – 5) = y 2 ⇒ 3 – x 5 = y 2 ⇒ –x 8 = y 2 ⇒ x

Selina Chapter 6 Linear Equations Including Problems Icse Solutions Class 9 Maths

Systems Of Equations With Elimination X 2y 6 4x 2y 14 Video Khan Academy

1234567891011Next